Chemical reaction kinetics: Basic principles
 An equilibrium reaction
An equilibrium reaction
We consider a unimolecular equilibrium reaction \[\text{A}\rightleftharpoons \text{B}\] between chemical species A and B. This actually involves two simultaneous reactions, namely
forward \(\displaystyle \text{A}\stackrel{k_1}{\longrightarrow} \text{B}\) with reaction rate constant \(k_1\)
backward \(\displaystyle \text{B}\stackrel{k_{2}}{\longrightarrow} \text{A}\) with rate constant \(k_{2}\)
Species A is not only converted into B, but it is also formed from species B by the reverse reaction. Similarly substance B is not only formed, but disappears again by the reverse reaction. When we assume elementary chemical reactions in this system, then the following two coupled differential equations describe the reaction kinetics: \[\left\{\begin{aligned}\frac{\dd[\text{A}]}{\dd t}&=-k_1[\text{A}]+k_{2}[\text{B}]\\ \\ \frac{\dd[\text{B}]}{\dd t}&=k_1[\text{A}]-k_{2} [\text{B}]\end{aligned}\right.\] Under the assumption that the total concentration of A and B is constant, we can uncouple the differential equations: if \([\text{A}]+[\text{B}]=K\) for some constant \(K\), then you can rewrite the equations as \[\begin{aligned}\frac{\dd[\text{A}]}{\dd t}&=-k_1 [\text{A}]+k_{2} (K-[\text{A}])\\ \\ \frac{\dd[\text{B}]}{\dd t}&=k_1 (K-[\text{B}])-k_{2}[\text{B}]\end{aligned}\] So: \[\begin{aligned}\frac{\dd[\text{A}]}{\dd t}&=k_{2} K-(k_1+k_{2}) [\text{A}]\\ \\ \frac{\dd[\text{B}]}{\dd t}&=k_1 K-(k_1+k_{2}) [\text{B}]\end{aligned}\] Both equations correspond with restricted exponential growth, and an equilibrium settles in after sufficient time.
There is chemical equilibrium when \[\frac{\dd[\text{A}]}{\dd t}=0, \frac{\dd[\text{B}]}{\dd t}=0\] The equilibrium concentrations \([\text{A}]_{\infty}\) and \([\text{B}]_{\infty}\) can be determined herewith; verify that the follwoing is true when the system is in equilibrium: \[[\text{A}]_{\infty}=\frac{k_{-1}\cdot c}{(k_1+k_{-1})},\quad [\text{B}]_{\infty}=\frac{k_{1}\cdot c}{(k_1+k_{-1})}\] In equilibrium, the concentrations are related as quotient of the forward and reverse reaction rate constants: \[\frac{[\text{A}]_{\infty}}{[\text{B}]_{\infty}} = \frac{k_{-1}}{k_{1}}\]
As a concrete example, we consider the reaction kinetics of the isomerization
\[\mathit{cis\mbox{-}}\mathrm{Mo(CO)}_4 \mathrm{[P(}\mathit{n}\,\mbox{-}\mathrm{Bu)}_3\mathrm{]}_2\rightleftharpoons \mathit{trans\mbox{-}}\mathrm{Mo(CO)}_4 \mathrm{[P(}\mathit{n}\,\mbox{-}\mathrm{Bu)}_3\mathrm{]}_2\] The reaction rate constants (in \(\mathrm{s}^{-1}\) ) are dependent on the temperature \(T\) (in Kelvin) according to the following formulas (Bengali and Mooney, 2003): \[k_1=T\cdot 10^{8.87-\frac{5195}{T}},\quad k_{-1}=T\cdot 10^{8.78-\frac{5394}{T}}\] The diagram below shows the time course of the concentrations of the cis- and trans-form (in uM) at a temperature of \(85{}^{\circ}\!\mathrm{C}\). Both curves match restricted exponential growth.

Below are shown the concentration curves at a temperature of \(80{}^{\circ}\!\mathrm{C}\). The previous concentration curves for \(85{}^{\circ}\!\mathrm{C}\) are in gray.
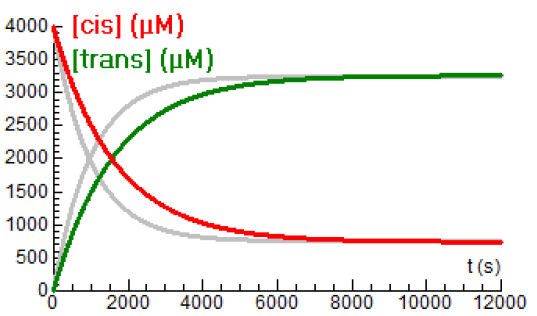
You can see in the above diagram that the equilibrium concentrations differ little, but that the equilibrium is reached slower at lower temperature. Apparently, the ratio of the rate constants remains almost equal.
\(\phantom{x}\)
Bengali, AA & Mooney, KE (2003). Synthesis, kinetics, and thermodynamics: an advanced laboratory investigation of the cis-trans isomerization of \(\mathrm{Mo(CO)}_4\mathrm{(PR}_3\mathrm{)}_2.\) Journal of Chemical Education 80(9), 1044-1047.


