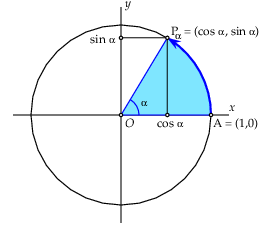
sin and cos Let \(A\) be the point \((1,0)\) on the unit circle \(C\) and define for any positive number \(\alpha\) the point \(P_{\alpha}\) on \(C\) as the point obtained by moving \(A\) anticlockwise along the rim of the unit circle over a distance \(\alpha\). The point \(P_{\alpha}\) has then by definition the coordinates \(\bigl(\cos(\alpha), \sin(\alpha)\bigr)\); for readability, we often omit some brackets and write \(\bigl(\cos\alpha, \sin\alpha\bigr)\). The figure below visualizes this definition. For any negative number \(\alpha\) a similar definition applies, but then the movement of the point \(A\) must be in the clockwise direction.

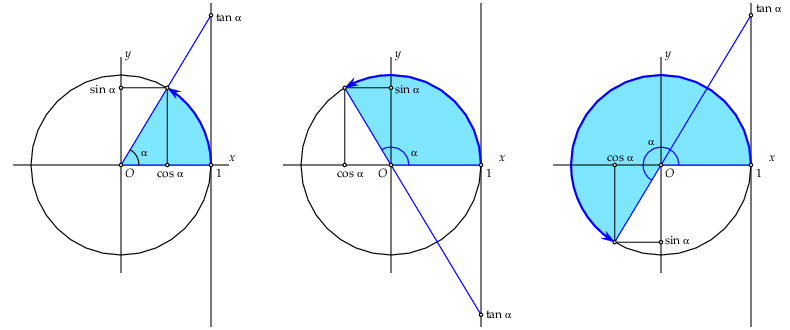
tan The tangent function can be defined as the quotient of the sine and cosine function, but can also be visualized using the unit circle. Draw a vertical line through the point \((1,0)\) and intersect the extended radius with that line. The \(y\)-coordinate of the intersection point is then \((1,\tan\alpha)\). Several cases for different values of \(\alpha\) are shown in the figures below.