Functions and graphs: Transformations of graphs and functions
Vertical translation
Vertical translation For any function \(f\) and any number \(d\), the graph of the function \(g\) defined by \(g(x)=f(x)+d\) is obtained from the graph of \(f\) by shifting it \(d\) upwards (if \(d<0\), then it is basically a downward vertical shift of the graph).
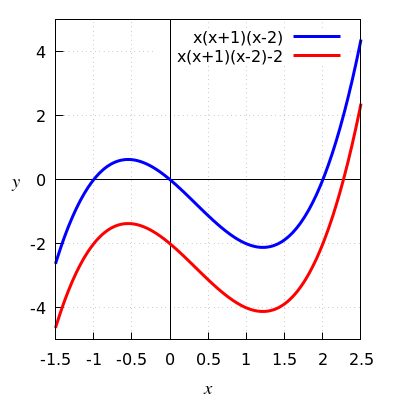
The figure below shows the graphs of the functions \(f(x)=x(x+1)(x-2)\) and \(g(x)=x(x+1)(x-2)-2\). So: \(g(x)=f(x)-2\).
You get the graph of \(g\) by moving that of \(f\) down by two.
Unlock full access
