Functions and graphs: Transformations of graphs and functions
Horizontal multiplication
Horizontal multiplication For any function \(f\) and any number \(b\neq 0\), the graph of the function \(g\) defined by \(g(x)= f(b\cdot x)\) is obtained from the graph of \(f\) by multiplying it horizontally by \(1/b\): the horizontal coordinate is multiplied by \(1/b\).
If \(b>0\), then it is about stretching or shrinking the graph relative to the vertical axis.
If \(b<0\), then all points will be on the other side of the vertical axis.
A special case is \(b=-1\): then the graph of \(f\) mirrored in the vertical axis.
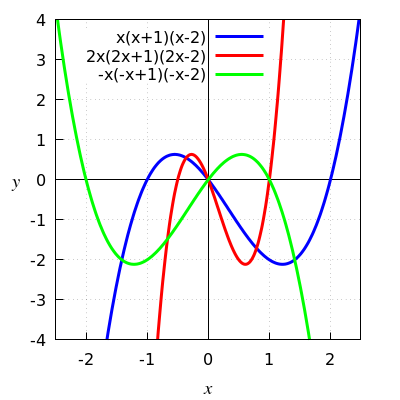
The figure below shows the graphs of \(x\mapsto x(x+1)(x-2)\), \(x \mapsto 2x(2x+1)(2x-2)\) and \(x \mapsto -x(-x+1)(-x-2)\).
You get the red graph of \(x \mapsto 2x(2x+1)(2x-2)\) by multiplying the blue graph of \(x\mapsto x(x+1)(x-2)\) horizontally by a factor of \(\frac{1}{2}\)
You get the green graph of \(x \mapsto -x(-x+1)(-x-2)\) by mirroring the blue graph of \(x\mapsto x(x+1)(x-2)\) in the vertical axis.
