A linear relationship on the basis of two data points

Basic functions: Linear functions

A linear relationship on the basis of two data points

You have already seen how the function definition of a linear function can be calculated if you are already known to the slope and a single point on the graph. In practice you encounter the following problem more frequently:

Given two data points $(t_0,y_0)$ and $(t_1,y_1)$ with $t_0\neq t_1$ , what is the function definition $y(t)=a\,t+b$ for which the graph goes through these two points?

The general solution method is as follows:

The graph of the linear function $y(t)=a\,t+b$ is a straight line.
The slope $a$ can be calculated as the quotient of increments:

$a=\frac{{\vartriangle}y}{{\vartriangle}t}=\frac{y_1-y_0}{t_1-t_0}$ Hereafter, the intercept $b$ can be calculated on the basis of the coordinates of one of the two data points, for example:

$b=y_0-a\cdot t_0$

A concrete dynamic example may illustrate the method.

Given two points $(3,3)$ and $(6,2)$ , what is the function rule $y(t)=a\,t+b$ for which the graph goes through these two points?

The graph of the linear function $y(t)=a\,t+b$ is a straight line.
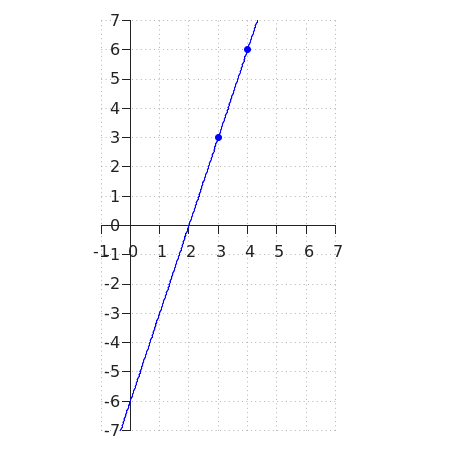
The slope $a$ can be calculated as the quotient of increments:

$a=\frac{{\vartriangle}y}{{\vartriangle}t}=\frac{2-3}{6-3}=-{{1}\over{3}}$ Hereafter, the vertical intercept $b$ can be calculated on the basis of the coordinates of one of the two measurement points, for example, on the basis of the point $(3,3)$ :

$3=-{{1}\over{3}}\times 3 +b\qquad\text{that\;is}\qquad b=3+{{1}\over{3}}\times 3=4$ The function definition is

$y(t)=-{{1}\over{3}}t+4$ The vertical intercept is $f(0)=b=4$ .
The graph of this function is shown along with the two data points in the figure below.

New example